Wie löse ich eine kubische Gleichung mit pgfmath?

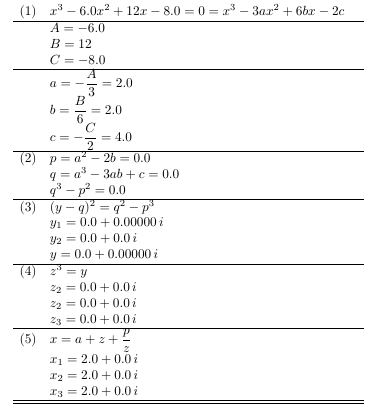
\documentclass[margin=5pt]{standalone}
\usepackage{amsmath, amssymb}
\usepackage{tikz}
%\usepackage{fp}
%\usetikzlibrary{fixedpointarithmetic}
%\pgfkeys{/pgf/fixed point arithmetic}
\begin{document}

% Beispiel  x³+Ax²+Bx+C=0

%% x^3 - 6 x^2 + 12 x - 8  =  (x-2)^3
\pgfmathsetmacro{\A}{-6} %  
\pgfmathsetmacro{\B}{12} % 
\pgfmathsetmacro{\C}{-8} %

%% x^3 - x^2 - x - 1 = 0 = schwieriges Ergeb.
%\pgfmathsetmacro{\A}{-1} %  
%\pgfmathsetmacro{\B}{-1} % 
%\pgfmathsetmacro{\C}{-1} %

%%% x^3-5x^2-4x+20 =(x-5)(x-2)(x+2)
%\pgfmathsetmacro{\A}{-5} %  
%\pgfmathsetmacro{\B}{-4} % 
%\pgfmathsetmacro{\C}{20} % 
%
%% x^3 - 6 x^2 + 11 x - 6    = (x-1)(x-2)(x-3)
%\pgfmathsetmacro{\A}{-6} %  
%\pgfmathsetmacro{\B}{11} % 
%\pgfmathsetmacro{\C}{-6} % 
%
%%% c^3−13c^2−21c+377= 0 geht nicht
%%\pgfmathsetmacro{\A}{-13} %  
%%\pgfmathsetmacro{\B}{-21} % 
%%\pgfmathsetmacro{\C}{377} % 
%
%% x^3-6.5 x^2-5.25 x+47.125 = 0
%\pgfmathsetmacro{\A}{-6.5} %  
%\pgfmathsetmacro{\B}{-5.25} % 
%\pgfmathsetmacro{\C}{47.125} %

% Berechnung
\pgfmathsetmacro{\Avz}{\A<0 ? "\A" : "+\A"}
\pgfmathsetmacro{\Bvz}{\B<0 ? "\B" : "+\B"}
\pgfmathsetmacro{\Cvz}{\C<0 ? "\C" : "+\C"}

\pgfmathsetmacro{\a}{-\A/3} %  
\pgfmathsetmacro{\b}{\B/6} %  
\pgfmathsetmacro{\c}{-\C/2} %

\pgfmathsetmacro{\p}{\a^2-2*\b} %  
\pgfmathsetmacro{\q}{\a^3-3*\a*\b+\c} % 
\pgfmathsetmacro{\D}{\q^2-\p^3} % 
\pgfmathsetmacro{\DTest}{\D > 0 ? 1 : 0} % 
\ifnum\DTest=1
\pgfmathsetmacro{\yIre}{\q+sqrt(\q^2-\p^3)} %  
\pgfmathsetmacro{\yIim}{0} %
\pgfmathsetmacro{\yIimVZ}{\yIim<0 ? "" : "+"}  
\pgfmathsetmacro{\yIIre}{\q-sqrt(\q^2-\p^3)} %  
\pgfmathsetmacro{\yIIim}{0} %  
\pgfmathsetmacro{\yIIimVZ}{\yIIim<0 ? "" : "+"}
\pgfmathsetmacro{\y}{"\yIre"}
%\pgfmathsetmacro{\y}{\yI >= 0 ? \yI : \yII} %  
\else%
\pgfmathsetmacro{\yIre}{\q} %  
\pgfmathsetmacro{\yIim}{sqrt(-\q^2+\p^3)} %  
\pgfmathsetmacro{\yIimVZ}{\yIim<0 ? "" : "+"}
\pgfmathsetmacro{\yIIre}{\q} %  
\pgfmathsetmacro{\yIIim}{-sqrt(-\q^2+\p^3)} %  -
\pgfmathsetmacro{\yIIimVZ}{\yIIim<0 ? "" : "+"}
\pgfmathsetmacro{\y}{"\yIre \yIimVZ \yIim \,i"}
\fi%

\pgfmathsetmacro{\yAbs}{sqrt((\yIre)^2+(\yIim)^2)} %  
\pgfmathsetmacro{\yW}{\yAbs^(1/3)} %  
\pgfmathsetmacro{\yArg}{atan2(\yIim,\yIre)} %

\pgfmathsetmacro{\zIre}{\yW*cos(\yArg/3)}  
\pgfmathsetmacro{\zIim}{\yW*sin(\yArg/3)}  
\pgfmathsetmacro{\zIimVZ}{\zIim<0 ? "" : "+"}
\pgfmathsetmacro{\zIabs}{sqrt((\zIre)^2+(\zIim)^2)}

\pgfmathsetmacro{\zIIre}{\yW*(-cos(\yArg/3)-sqrt(3)*sin(\yArg))/2}  
\pgfmathsetmacro{\zIIim}{\yW*(sqrt(3)*cos(\yArg/3)-sin(\yArg))/2}
\pgfmathsetmacro{\zIIimVZ}{\zIIim<0 ? "" : "+"}
\pgfmathsetmacro{\zIIabs}{sqrt((\zIIre)^2+(\zIIim)^2)}

\pgfmathsetmacro{\zIIIre}{\yW*(-cos(\yArg/3)+sqrt(3)*sin(\yArg))/2}
\pgfmathsetmacro{\zIIIim}{\yW*(-sqrt(3)*cos(\yArg/3)-sin(\yArg))/2}
\pgfmathsetmacro{\zIIIimVZ}{\zIIIim<0 ? "" : "+"}
\pgfmathsetmacro{\zIIIabs}{sqrt((\zIIIre)^2+(\zIIIim)^2)}

\pgfmathsetmacro{\pTest}{\p==0 ? 1 : 0}
\ifnum\pTest=1%
\pgfmathsetmacro{\xIre}{\a + \zIre}%
\pgfmathsetmacro{\xIim}{\zIim}%
\pgfmathsetmacro{\xIimVZ}{\xIim<0 ? "" : "+"}%
%
\pgfmathsetmacro{\xIIre}{\a+\zIIre}%
\pgfmathsetmacro{\xIIim}{\zIIim}%
\pgfmathsetmacro{\xIIimVZ}{\xIIim<0 ? "" : "+"}%
%
\pgfmathsetmacro{\xIIIre}{\a+\zIIIre}%
\pgfmathsetmacro{\xIIIim}{\zIIIim}%
\pgfmathsetmacro{\xIIIimVZ}{\xIIIim<0 ? "" : "+"}%
\else%
\pgfmathsetmacro{\xIre}{\a+\zIre*(1+\p/\zIabs^2)}%
\pgfmathsetmacro{\xIim}{\zIim*(1-\p/\zIabs^2)}%
\pgfmathsetmacro{\xIimVZ}{\xIim<0 ? "" : "+"}%
%
\pgfmathsetmacro{\xIIre}{\a+\zIIre*(1+\p/\zIIabs^2)}%
\pgfmathsetmacro{\xIIim}{\zIIim*(1-\p/\zIIabs^2)}%
\pgfmathsetmacro{\xIIimVZ}{\xIIim<0 ? "" : "+"}%
%
\pgfmathsetmacro{\xIIIre}{\a+\zIIIre*(1+\p/\zIIIabs^2)}%
\pgfmathsetmacro{\xIIIim}{\zIIIim*(1-\p/\zIIIabs^2)}%
\pgfmathsetmacro{\xIIIimVZ}{\xIIIim<0 ? "" : "+"}%
\fi%

$\begin{array}{c l r}
(1) &  x^3 \Avz x^2 \Bvz x \Cvz = 0   
= x^3 -3a x^2 + 6b x - 2c  \\ \hline
& A = \A & \\
& B = \B & \\
& C = \C  & \\    \hline
& a = -\dfrac{A}{3} = \a \\
& b = \dfrac{B}{6} = \b \\
& c = -\dfrac{C}{2} = \c \\  \hline
(2) & p = a^2-2b = \p \\
& q = a^3-3 ab+c = \q \\ 
& q^3-p^2 = \D \\    \hline
(3) & (y-q)^2 = q^2-p^3 \\
& y_1 = \yIre \yIimVZ \yIim \,i   \\
& y_2 = \yIIre \yIIimVZ \yIIim \,i   \\
& y=\y \\ \hline
(4) & z^3 = y \\ 
& z_2 = \zIre \zIimVZ \zIim \,i \\
& z_2 = \zIIre \zIIimVZ \zIIim \,i \\
& z_3 = \zIIIre \zIIIimVZ \zIIIim \,i  \\ \hline
(5) & x = a + z + \dfrac{p}{z} \\
& x_1 = \xIre  \xIimVZ    \xIim \,i \\
& x_2 = \xIIre  \xIIimVZ    \xIIim \,i \\
& x_3 = \xIIIre \xIIIimVZ \xIIIim \,i       \\ \hline\hline
\end{array}$

\end{document}